Detection of terrestrial planets and moons with the photometric transit method
Hans J. Deeg
Instituto de
Astrofísica de Canarias, C. Via Lactea s/n, E-38200 La Laguna, Tenerife, Spain,
Email: hans.deeg@iac.es
ABSTRACT
An overview is given over the photometric transit method for the detection of extraterrestrial planets. Discussed are: its basic principals, its derivable parameters, requirements for its implementation, and sources of confusion. Special emphasis is given space based experiments that may lead towards the detection of Earth-like planets. The detection of large moons may also be possible, either by their direct detection in the shape of a transit lightcurve, or by their influences on the mid-time of transit. For the latter point, estimations are given on the potential that will offer the upcoming three space missions, COROT by CNES, Kepler by NASA and Eddington by ESA for the detection of moons around extrasolar planets. By a similar principle, measurements of variations in the exact minimum time of eclipsing binaries are also expected to lead to a large number of detections of circumbinary planets.
1. Introduction
The possible existence of other worlds – that is, Earth-like planets - has been a source of inspiration for astronomers and lay-persons alike for centuries. While the large number of discoveries of extrasolar planets in the last few years [[1]] has been an enormous step in that direction, the ‘radial velocity’ method that is employed for these discoveries has fundamental limits that will not allow the discovery of planets smaller than about 10 Earth masses.
An alternative that has received increasing attention in recent years is the detection of planetary transits, where a planet crosses in front of its central star. By an external observer this is perceived as a slight dimming of the star that is being crossed. This ‘transit method’ was mentioned first already 50 years ago`[[2]*], though the first serious outline of the method did not appear until 1971 [[3]]. Further developments during the 1980’s and 90’s [[4],[5],[6]] culminated in the first proposal for a dedicated space mission[[7]].
Especially since the discovery of the first transiting planet in 1999 [[8],[9]], several ground based transit projects have been initiated, which are however mainly dedicated to the detection of short periodic ‘Hot Jupiters’ (for an overview, see [[10]]). Of more interest for the purpose of this article are however the experiments dedicated towards the detection of Earth-like planets. Incidentally, the first experiment employing the transit method was dedicated to just this. This was the TEP (Transits of Extrasolar Planets) project [[11],[12]], in which the eclipsing binary star CM Draconis was surveyed during the years 1994-2000 for the presence of large terrestrial circumbinary planets, reaching a detection limit of 2.5 Earth diameters. Further ground based experiments towards Earth-like planet detection around M stars exist currently only as proposals, but several space missions are now approved for launch in the coming years. These should make the discovery of true Earth equivalents (in terms of size and distance from the central star, which also implies a temperature similar to Earth) around Solar-like stars possible until the end of the decade with the method of transits.
2. Basic principles
.

Fig.
1. Basic principle of the transit method. A planet transiting in front of its
own central star will cause a small dip in the stellar brightness L*.
d is the transit’s latitude
on the stellar disk.
The basic idea of the transit method is shown in Fig. 1. The brightness variation during a transit, DL, and the star's off-transit brightness L*, are approximately related to the radii of the planet and star by:
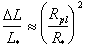 (1)
(1)
Equation (1) assumes a uniform surface brightness of the transited star. The exact shape of the brightness drop depends also on the latitude of the transit across the central star, and on the stellar limb-darkening, For a star with a limb darkening coefficient of 0.5, the maximum of the brightness drop is about 20% larger. Since the stellar limb-darkening is strongly dependent on the wavelength, a planetary transit event will also cause a characteristic, albeit small, color-change. If we assume the Earth-Sun system as typical, the observed brightness variation of an Earth-like planet would be about DL/L = 8.4 . 10-5. This brightness variation is much too weak to be detectable from ground based observatories, where limits are about one part in a thousand. This is the principal requirement that causes the need for space missions. Ground-based transit detections of terrestrial planets will therefore only be possible around M stars, which are smaller, and consequently, transits are deeper by an order of magnitude.
The duration of a transit, ttr, is given by:
 , (2)
, (2)
where d is the latitude of the transit on the stellar disk, apl is the major halfaxis of the planetary orbit, and Tpl is the orbital period. Again for the Earth-Sun system, ttr for an external observer would be about 13 hours. The period Tpl can trivially be determined if several transits at time intervals Tpl are observed. In actual observing programs, the observation of several – at least three – transits is desirable, as the repeatability is a key feature to verify observed brightness drops as planetary transits. This requirement also makes space mission very attractive, since only they can ascertain observations over several planetary orbital periods without significant interruptions. A detailed example on the derivation of the various planetary and stellar parameters from ground based observations of a transit of HD209458 is given in ref. [[13]].
In order to produce transits, it is of course necessary that a planet-star system orbits in a plane that is within a small angle to the line of sight. Or, as seen from the perspective of the central star, from anywhere within the band in the sky that is subtended by its planet during one orbit, a transit can be observed. The probability for a randomly oriented planet-star system to produce transits is then given by:
ptr = R*/apl (3)
For the Earth-Sun system, this probability is 0.47%. Considering that Venus is another Earth-like planet with similar size, and its probability for alignment is 0.65%, the total probability for an external observer to detect any Earth-like planet around the Sun is about 1%. The probability to detect short periodic planets is of course larger, and in the case of Hot Giant planets it reaches over 5%. Another selection effect is given by the stellar populations that are being observed. Only main sequence stars are of interest in transit surveys, but they account only for about half of the stars within the brightness limits of most transit searches. Giant stars are too big and photometrically unstable to produced observable planetary transits. Thus, not more than one in 200 field stars can be expected to show transits of Earth-like planets – this if Solar Systems equivalents are very common. Consequently, thousands of stars need to be observed, causing the need for wide-field photometric cameras transit surveys.
Lastly, the problem of unequivocal detections of transiting planets should be mentioned. This becomes especially concerning in situations were the transit signal is relatively weak. The two principal issues here are: stellar micro variability causing transit like signatures, and eclipsing binaries masking as planet-star systems.
Regarding the first point, it has been demonstrated [[14]] that the two phenomena’s different frequency domains allow in their separation, and hence variability is not expected to be a major obstacle in Earth-like planet detection. Also, the difference in colors between microvariability and transits will allow their discrimination where multi-color data are available.
Another source of confusion will be eclipsing binary (EB) stars. A grazing EB has a low-amplitude eclipse that may appear like a transit of a planet. Or, an EB with a large amplitude in the background of a brighter star** could give a low amplitude signal in the summed light. These cases may be discriminated against transits by considering their period and duration, their shape, and their color, if such data are taken. Follow-up radial velocity observations would also reveal grazing EB’s, whereas optical data with high spatial resolution may resolve background EB’s. Some number of false positives in transits of weak signal may however be unavoidable.
3. Space missions for the detection of terrestrial planets
Table 1: Overview on transit space projects
|
Name |
COROT |
Kepler |
Eddington |
|
Organization |
CNES
(F) + partners |
NASA |
ESA |
|
Launch
date |
2005 |
2007 |
2007-8 |
|
Mission
duration |
3
yrs |
4
yrs |
5yrs
(3 yrs for EP) |
|
Dedication |
AS
+ EP (parallel
obs) |
EP |
AS+EP
(sequential obs) |
|
Telescope Æ |
20 cm |
95 cm |
4 x
60cm* |
|
Field
of view |
4
deg2 (EP) |
105
deg2 |
36
deg2 * |
|
Color-Bands |
3 |
1 |
1-4*
|
|
Nr.
of stars obsv’d for EP |
12
k |
100
k |
50
– 100 k |
* Preliminary or still under
discussion
Three space missions are currently in preparation, and all are approved for launches between 2005 and 2008 (Table 1). The first one is the french-led COROT mission[[15],[16]], which contains a small 20cmØ telescope dedicated to asteroseismology (AS) and extrasolar planet (EP) detection COROT will take AP and EP observations simultaneously in stellar fields that are side-by-side in the sky, with two sets of CCDs, each one optimized to its specific task. With COROT’s maximum observing duration of 5 months on any single field, only short periodic planets will cause 3 observable transit events. Therefore, a prism that gives 3-color photometry has been added, with the intention to recognize planets from observations of single transit events, due to the unique color signature of transits. COROT has therefore some sensitivity to longer periodic planets as well, albeit less information will be gained on these. Two larger space missions are then foreseen for the years 2006-8 - Kepler by NASA and Eddington by ESA. Kepler[[17][18]] is the only mission dedicated exclusively to the detection of Earth-like planets. It will observe 100 000 K dwarf stars in a single large field in Cygnus over 4 years. Lastly, ESA approved recently (May 2002) the mission Eddington[[19][20]] for a launch not later than 2008. Current studies for Eddington center on a design with 4 Schmidt telescopes of about 60cm diameter. Observations in AS and EP are to be taken sequentially, with 2 years dedicated to AS and 3 yrs to EP, though of course observations for one topic may benefit the other. Like COROT, Eddington will probably employ some form of multi-color photometry. In Eddington’s case this is however motivated by the improved discrimination against false positives, as mentioned in the previous section. The number and wavelengths of colors bands in Eddington is still to be determined. In Fig. 2 an overview is given on the expected planet detection capabilities of these space missions.
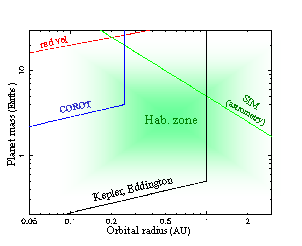
Fig. 2. Masses and orbital distances of planets that are accessible for detection by the transit space mission, in comparison to ground based radial velocity measurements and the SIM proposal for astrometric planet detection. The habitable zone in terms of planet mass and distance is given for a solar-like star.
4. Analysis of planetary atmospheres
During a transit, a planet’s atmosphere will appear as a partially opaque ring for an external observer. The scale height of this ring is of course wavelength dependent, giving therefore the planet an effective radius that is a function of wavelength. Precise transit observations with spectroscopes - comparing transit depth across different wavelengths- or by photometers with filters may therefore give an indication about a planet’s atmospheric composition. Studies on the effects of Hot Giant planet atmospheres onto transits have been published by [[21],[22],[23]], and a possible absorption by sodium from the planet around HD209458 has been reported [[24]] from observations with the Hubble Space Telescope’s STIS spectrograph. In that case, the transit at the sodium feature’s wavelength is reported to be about 2 x 10-4 times deeper than in adjacent bands. This small value makes it clear that detection of atmospheric features around terrestrial planets is going to become a very difficult task. Additionally, the detection of wide-band features -or colors- is complicated by the color dependency of transits depths and shapes due to the wavelength dependent stellar limb darkening [11,[25]].
An inquiry has been made if O2 -an indicator for potential life- could be detected in transiting Earth like planets [[26]]. The result is that 8m class telescopes could detect the O2 A-band absorption feature for such planets around main-sequence M stars of 10th magnitude. There are only few M stars with that brightness, but with significantly larger telescopes, or future improved instrumentation, O2 detections may be extended to M stars of 13th magnitude.
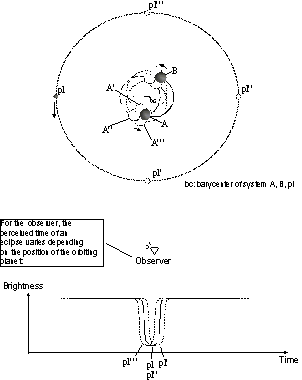
Fig.
3. Schematics of the movement of a binary star orbit under the influence of an
orbiting planet, and its effect on eclipse minimum times. Depending on the
position of the planet (pl, pl', pl'', pl'''), the binary components will be at
shifted orbits (A, A', A'',A''' for component A), and the variation in the
distance to the observer will be perceived as on offset in the time of the
eclipse minima from strict periodicity.
5. timing of transit observations - possibilities for further detections
Special consideration we give here to the results that may be achievable from the precise timing of eclipse-like features in a lightcurve - be it an 'eclipse' of a binary star system, or a 'transit' from a star-planet system. In both cases, an unseen third body will cause the eclipsing system to be offset from the 3-body barycenter. The precision dto of the measurement of the time t0 of minimum light in a lightcurve dominated by white Gaussian noise can be estimated as follows:
 (4)
(4)
where L is the flux from the object at
discrete times ti (the
lightcurve) and dL the relative
error in the measurement of L. For lightcurves with equidistant
points ti, where Dt=ti+1 – ti, and with the relation against a variation in the minimum time t0:
![]() , a simple calculation of the derivative of Eq.(4) is
possible from a single lightcurve, with
, a simple calculation of the derivative of Eq.(4) is
possible from a single lightcurve, with
![]() . (5)
. (5)
As an example from ref. [[27]] (note that Eq. 3 in that reference contains an error and is corrected by above Eq. 4), the precision in minimum timing measurements of the eclipsing binary CM Dra in terrestrial observations is about 1.5 seconds, assuming a relative photometric error of dB = 0.01 and a sample time of Dt = 5 sec.
5.1 Planets around eclipsing binaries
Any wide field photometric survey for the detection of transits will unavoidably detect a substantial number of eclipsing binaries (EBs). Previously, EBs were mentioned as an undesirable source of confusion against real planetary transits - they do however also offer a further way to detect planets. Around close binaries there are stable orbits for planets if their half axis exceeds about 3 times the binary components’ separation. Orbiting planets are then offsetting the binary star around the barycenter common to all 3 bodies. Consequently, the variations in light travel time from the binary will then make its eclipses appear earlier or later, with a periodicity given by the third body's orbital period (Fig. 3). The amplitude of the timing offset Dt0 is given by equation (6):
![]() , (6)
, (6)
were Mpl and M*
are the masses of planet and star, and c
is the speed of light. It is noteworthy, that these planet detections do not require the presence of planetary
transits. However, similarly to the radial velocity method, the planet’s mass
can only be given as m sin i, where i is the planet’s orbital inclination. For planets around an EB it
can however be expected that they orbit close to the EB’s orbital plane, which
is always close to i = 90°, and hence sin i is also
close to 1.
Observations of eclipse minima with a timing precision of about 6 seconds have already led to the establishment of a lower limit of 1-3 Jupiter masses for the presence of long periodic planets (500-2000 days; the mass limit is dependent on the orbital period) around the EB system CM Draconis [[28]]. The upcoming space missions will of course provide much lower detection limits, and due to their photometric precision, sub-second minimum timing precision can be expected from them (Fig. 4). The lower limits for planet detection with these missions will be given by the brightest EB’s they can observe without saturation (11-12th mag for Eddington).
5.2 Detection of Moons
With precise timing, planetary transits may
also indicate the presence of moons around their planets. For one, a large moon
may be visible directly in the shape
of a transit [[29]]. This can
be expected, if the ratio in sizes between moon and planet is not a great one.
Another 
effect is the deviation of the center time of a transit from
strict periodicity, as the planet -which will cause the principal transit- will
be offset from the planet-moon barycenter, and may lead, or trail the
barycenter in individual transits. The observation of repeated transits with
high timing precision may therefore indicate the presence of moons. As an
example, the Earth leads or trails the Earth-Moon barycenter on its orbit
around the Sun by up to 2.5 minutes. Similarly, Saturn is offset by its largest
moon, Titan, by up to 30 seconds. Whereas the ratio in cross sections between
Titan and Saturn is about 1:550 and hence a direct transit signal from a
Titan-like moon cannot be expected to be observable, future space mission may
well be able to detect offsets in transit times by 30 seconds (Fig. 5), hence
leading to the expectation that some Galilean-like moons may be detected. Such
a detection will of course need at least 3, but preferably more minimum-timing
measurements to establish a periodicity in the timing offsets. With the space
missions Kepler and Eddington, and their multi-year observing runs, moons
around planets with orbital periods of up to 200days may however well be
detectable.
6. Summary
Three upcoming space missions, -all of them approved for launch before 2008- lead to the expectation, that a significant number of Earth-like, and more general, terrestrial planets will known within a decade. The possibility to obtain precise timings of eclipses from planetary transits and eclipsing binaries alike gives the opportunity to detect large moons around giant planets in the first case, and to detect non-transiting planets around close binary stars for the seconds case. Both of these possibilities may significantly enhance the scientific return of these space missions.
REFERENCES